A simple and conservative empirical likelihood function

Guthrie Miller

Multiple measurements with an unknown Gaussian likelihood function are treated probabilistically. The likelihood function

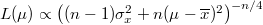$L(\mu)\propto \left( (n-1)\sigma_x^2 +n(\mu-\overline{x})^2 \right)^{-n/4}$ is derived

where 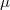$\mu$ is the true value, 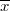$\overline{x}$ is the mean,

and 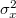$\sigma_x^2$ is the variance obtained from the 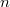$n$ measurements.

DOI Code: 10.1285/i20705948v7n2p432

Keywords: likelihood function, empirical, data analysis, lognormal, probabilistic, Bayesian.

Sivia, D. S. with Skilling, J. (2006). Data Analysis--A Bayesian Tutorial, 2nd Ed. Oxford Science Publications.

Guthrie Miller. (2013) Probabilistic Interpretation of Data--A Physicist's Approach. Lulu Publications.

Full Text: pdf

کاغذ a4

Electronic Journal of Applied Statistical Analysis