Sequences of ideal norms
Abstract
There is a host of possibilities to associate with every (bounded linear) operator T, acting between Banach spaces, a scalar sequence 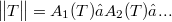 such that all maps
such that all maps 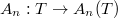 are ideal norms. The asymptotic behaviour of
are ideal norms. The asymptotic behaviour of 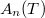 as
as  can be used to define various subclasses of operatore. The most simple condition is that
can be used to define various subclasses of operatore. The most simple condition is that 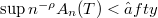 where
where 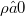 . Tris yiehis a 1-parameter scale of Banach operator ideals. In what follows, this construction will be applied in some concrete cases. In particular, we let
. Tris yiehis a 1-parameter scale of Banach operator ideals. In what follows, this construction will be applied in some concrete cases. In particular, we let 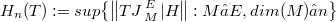 where J{E \atop M} denotes the canonical embedding from the subspace M into E. Note that
where J{E \atop M} denotes the canonical embedding from the subspace M into E. Note that 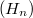 is the natural dimensional gradation of the Hilbertian operator norm
is the natural dimensional gradation of the Hilbertian operator norm 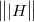 in the sense of A. Pelczynski ([30], p. 165) and N. Tomczak-Jägermann ([46] and [48], p. 175). Taking the infimum over all
in the sense of A. Pelczynski ([30], p. 165) and N. Tomczak-Jägermann ([46] and [48], p. 175). Taking the infimum over all 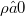 with
with 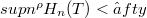 we get an index
we get an index ![h(T) ⊂ [0,1/2]](http://siba-ese.unisalento.it/plugins/generic/latexRender/cache/3f64110714a7813de02ccfdb77b5f74d.png) which can be used to measure the <<Hilbertness>> of the operator T. Our main purpose is to show that several sequences of concrete ideal norms have the same asymptotic behaviour. This solves a problem posed in ([48],p. 210). We also give some applications to the geometry of Banach spaces. Conceming the basic definitions and various results from the theory of operator ideals, the reader is referred to my monographs [31] and [32]. The notation is adopted from the latter. The present paper is a revised and extended version of my preprint [36]. This revision became necessary when I observed that its main result was already contained in Remark 13.4 of G. Pisier's book [43]; see 5.3 below.
which can be used to measure the <<Hilbertness>> of the operator T. Our main purpose is to show that several sequences of concrete ideal norms have the same asymptotic behaviour. This solves a problem posed in ([48],p. 210). We also give some applications to the geometry of Banach spaces. Conceming the basic definitions and various results from the theory of operator ideals, the reader is referred to my monographs [31] and [32]. The notation is adopted from the latter. The present paper is a revised and extended version of my preprint [36]. This revision became necessary when I observed that its main result was already contained in Remark 13.4 of G. Pisier's book [43]; see 5.3 below.
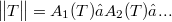 such that all maps
such that all maps 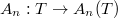 are ideal norms. The asymptotic behaviour of
are ideal norms. The asymptotic behaviour of 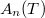 as
as  can be used to define various subclasses of operatore. The most simple condition is that
can be used to define various subclasses of operatore. The most simple condition is that 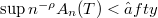 where
where 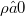 . Tris yiehis a 1-parameter scale of Banach operator ideals. In what follows, this construction will be applied in some concrete cases. In particular, we let
. Tris yiehis a 1-parameter scale of Banach operator ideals. In what follows, this construction will be applied in some concrete cases. In particular, we let 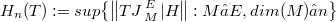 where J{E \atop M} denotes the canonical embedding from the subspace M into E. Note that
where J{E \atop M} denotes the canonical embedding from the subspace M into E. Note that 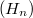 is the natural dimensional gradation of the Hilbertian operator norm
is the natural dimensional gradation of the Hilbertian operator norm 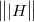 in the sense of A. Pelczynski ([30], p. 165) and N. Tomczak-Jägermann ([46] and [48], p. 175). Taking the infimum over all
in the sense of A. Pelczynski ([30], p. 165) and N. Tomczak-Jägermann ([46] and [48], p. 175). Taking the infimum over all 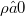 with
with 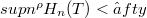 we get an index
we get an index ![h(T) ⊂ [0,1/2]](http://siba-ese.unisalento.it/plugins/generic/latexRender/cache/3f64110714a7813de02ccfdb77b5f74d.png) which can be used to measure the <<Hilbertness>> of the operator T. Our main purpose is to show that several sequences of concrete ideal norms have the same asymptotic behaviour. This solves a problem posed in ([48],p. 210). We also give some applications to the geometry of Banach spaces. Conceming the basic definitions and various results from the theory of operator ideals, the reader is referred to my monographs [31] and [32]. The notation is adopted from the latter. The present paper is a revised and extended version of my preprint [36]. This revision became necessary when I observed that its main result was already contained in Remark 13.4 of G. Pisier's book [43]; see 5.3 below.
which can be used to measure the <<Hilbertness>> of the operator T. Our main purpose is to show that several sequences of concrete ideal norms have the same asymptotic behaviour. This solves a problem posed in ([48],p. 210). We also give some applications to the geometry of Banach spaces. Conceming the basic definitions and various results from the theory of operator ideals, the reader is referred to my monographs [31] and [32]. The notation is adopted from the latter. The present paper is a revised and extended version of my preprint [36]. This revision became necessary when I observed that its main result was already contained in Remark 13.4 of G. Pisier's book [43]; see 5.3 below.DOI Code:
10.1285/i15900932v10supn2p411
Full Text: PDF


