A strict topology for some weighted spaces of continuous functions
Abstract
In the classical case the strict topology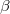 
introduced by Buck [2] on the space 
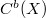of bounded continuous scalar valued functions on the locally compact Hausdorff space X is given by the system W of all weights on X that vanish at infinity. The 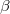-bounded subsets of 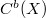are exactly the norm bounded subsets, and 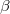is the finest locally convex topology which coincides on the norm bounded subsets with the compact open topology (cf. Dorroh [4]). Especially we have that 
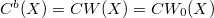holds algebraically. In this note we want to describe for an arbitrary system of weights V an associated system of weights W such that at least in many cases, including the classical one, the connection between 
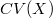and 
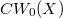is the same as in the classical case.
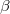introduced by Buck [2] on the space 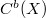of bounded continuous scalar valued functions on the locally compact Hausdorff space X is given by the system W of all weights on X that vanish at infinity. The 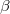-bounded subsets of 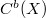are exactly the norm bounded subsets, and 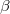is the finest locally convex topology which coincides on the norm bounded subsets with the compact open topology (cf. Dorroh [4]). Especially we have that 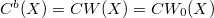holds algebraically. In this note we want to describe for an arbitrary system of weights V an associated system of weights W such that at least in many cases, including the classical one, the connection between 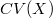and 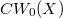is the same as in the classical case.DOI Code:
10.1285/i15900932v11p135
Full Text: PDF
