Flokki planes and cubic polynomials
Abstract
Non-Desarguesian translation planes of order 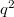 are constructed whenever
are constructed whenever  and
and  isnot divisible by
isnot divisible by 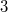 . Each plane has kernel
. Each plane has kernel 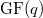 andtranslation complement of order
andtranslation complement of order 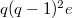 , with orbits of lengths
, with orbits of lengths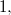
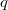 and
and 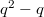 on the translation line. The planes have elation groups oforder
on the translation line. The planes have elation groups oforder 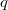 that producederivable nets, but are not flock planes, semifield planes, orlifted planes.
that producederivable nets, but are not flock planes, semifield planes, orlifted planes.
The same algebraic tools are used to construct non-Desarguesiantranslation planes of order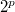 for every prime
for every prime 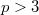 .
.
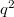 are constructed whenever
are constructed whenever  and
and  isnot divisible by
isnot divisible by 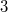 . Each plane has kernel
. Each plane has kernel 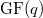 andtranslation complement of order
andtranslation complement of order 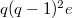 , with orbits of lengths
, with orbits of lengths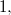
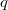 and
and 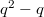 on the translation line. The planes have elation groups oforder
on the translation line. The planes have elation groups oforder 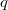 that producederivable nets, but are not flock planes, semifield planes, orlifted planes.
that producederivable nets, but are not flock planes, semifield planes, orlifted planes.The same algebraic tools are used to construct non-Desarguesiantranslation planes of order
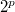 for every prime
for every prime 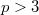 .
.DOI Code:
10.1285/i15900932v29n1supplp211
Keywords:
translation planes
translation planes
Full Text: PDF


