Uno studio delle equazioni di evoluzione ottenute dalle equazioni di Navier-Stokes discretizzando la parte spaziale
Abstract
Many Authors study the evolution equations discretising either the space or the time coordinates and solving then the ordinary differential equations obtained.A review paper where a large bibliography is shown is the one written by Liskovets [1].In the mathematical physics some authors, both for general evolution problems [2] and for specific problems [3] have prefered to discretize the time variable obtaining in this way a system ordinary differential equations with boundary conditions.In this way the difficulties arising when non stationary problems are treated by discretising both the time and space variable, are attenued. In this paper we prefer to discretize only the space variable and study a Cauchy problem for Navier-Stokes equations where, by introducing an auxiliary function 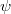 ,we avoid to treat separately the Poisson problem for the pressure.
,we avoid to treat separately the Poisson problem for the pressure.
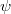 ,we avoid to treat separately the Poisson problem for the pressure.
,we avoid to treat separately the Poisson problem for the pressure.DOI Code:
10.1285/i15900932v3n1p117
Full Text: PDF


