Products of groups and local nearrings
Abstract
Groups which can be written as a product  of two of its subgroups
of two of its subgroups 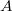 and
and 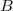 have been studied by many authors. A particular role in such investigations play groups of the form
have been studied by many authors. A particular role in such investigations play groups of the form 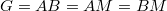 where
where  is a normal subgroup of
is a normal subgroup of  and
and 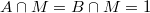 . It turns out that there is a close connection between groups of this form and some nearrings, especially so called local nearrings, so that many problems concerning such nearrings can be reduced to questions about these groups. In the following we will discuss different aspects of this connection and, in addition, consider in detail certain structural questions arised in the case of almost cyclic subgroups
. It turns out that there is a close connection between groups of this form and some nearrings, especially so called local nearrings, so that many problems concerning such nearrings can be reduced to questions about these groups. In the following we will discuss different aspects of this connection and, in addition, consider in detail certain structural questions arised in the case of almost cyclic subgroups 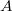 an
an 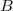 .
.
DOI Code:
10.1285/i15900932v28n2supplp177
Keywords:
factorized group; derivation; local nearring; multiplicative group
factorized group; derivation; local nearring; multiplicative group
Full Text: PDF


