On the mathematical work of Klaus Floret
Abstract
In section 1 of this article, a sketch of the life and the career of Klaus Floret is given. Then,in sections 2 to 5, the author reports on the part of the mathematical work of Klaus Floret which was devoted to four important topics in functional analysis in which Klaus worked and in which considerable progress was achieved during his lifetime. These topics are: locally convex inductive limits; bases and approximation properties; 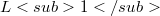 -
- 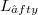 -spaces and the "probleme des topologies"; tensor norms, operator ideals, spaces of polynomials. Some of the history of these topics is included, sometimes together with an account of some of the leading figures in the field. A prelude, an interlude and a coda present related remarks which do not fit in the main body of the article.
-spaces and the "probleme des topologies"; tensor norms, operator ideals, spaces of polynomials. Some of the history of these topics is included, sometimes together with an account of some of the leading figures in the field. A prelude, an interlude and a coda present related remarks which do not fit in the main body of the article.
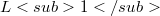 -
- 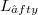 -spaces and the "probleme des topologies"; tensor norms, operator ideals, spaces of polynomials. Some of the history of these topics is included, sometimes together with an account of some of the leading figures in the field. A prelude, an interlude and a coda present related remarks which do not fit in the main body of the article.
-spaces and the "probleme des topologies"; tensor norms, operator ideals, spaces of polynomials. Some of the history of these topics is included, sometimes together with an account of some of the leading figures in the field. A prelude, an interlude and a coda present related remarks which do not fit in the main body of the article.DOI Code:
10.1285/i15900932v25n1p1
Keywords:
Curriculum vitae of Klaus Floret; List of publications of Klaus Floret; Locally convex inductive limit; Basis; Approximation property; $L1$-space; $L∞$-space; Problème des topologies; Tensor norm; Operator ideal; Spaces polynomials
Classification:
01A70; 46-02; 46A04; 46A11; 46A13; 46A32; 46A35; 46A50; 46B07; 46B15; 46B22; 46B28; 46G20; 46G25; 46M05; 46M40
Full Text: PDF


